|
Different uses for voting
need different types of voting. |
 |
Data on Manipulability |
 |

|
All decisive, non-dictatorial voting systems can be manipulated, sometimes. The operant questions are 'How often is each voting system manipulable in a realistic electorate, how easy is the manipulation, and how damaging is its effect?'[1] The evidence here shows Condorcet-Hare hybrids (C-IRV) resist manipulation best. They are even better than the usual majority IRV (M-IRV).
The voting rules discussed here were defined on the Other Rules page in the chapter about single-winner elections. In 2010, James Green-Armytage at the University of California Santa Barbara published Strategic Voting and Nomination. “Abstract: Using computer simulations based on three separate data generating processes, I estimate the fraction of elections in which sincere voting will be a core equilibrium given each of eight single-winner voting rules. Additionally, I determine how often each voting rule is vulnerable to simple voting strategies such as ‘burying’ and ‘compromising’, and how often each voting rule gives an incentive for non-winning candidates to enter or leave races. I find that Hare is least vulnerable to strategic voting in general, whereas Borda, Coombs, approval, and range are most vulnerable. I find that plurality is most vulnerable to compromising and strategic exit, and that Borda is most vulnerable to strategic entry. I support my key results with analytical proofs.” This confirms and extends the findings of John Chamberlin et al at the University of Michigan, and of Samuel Merrill III at Wilkes University. The key tables from those earlier studies are shown below with the authors' permissions. Punishing the leading candidate with last-place votesMost voting rules reward opposition voters for “punishing” the leading candidate with last-place votes. That usually hurts the leader's score, which helps the opposition's favorite candidate to win.In contrast, punishing the leading candidate with a last-place vote cannot help the voter's first choice to win under Condorcet's rule. The voter already ranks his favorite as number one. So an insincere ballot cannot increase the number of voters who rank his favorite, B, ahead of the main rival, A. But the punishing vote might decrease the chance that A could win by Condorcet's rule, because the insincere voter might be helping another candidate, C, (whom he would rank below both A and B on a sincere ballot) to beat the original leader. This may make C win by Condorcet's rule or it may create a voting cycle. In fact, even if most voters would honestly rank C last, insincere ballots can sometimes make her a Condorcet winner. Systems that reward punishing votes are less likely to find true Condorcet winners. I have adapted this example from one Merrill (on page 66) used to prove that Condorcet-completion rules do not necessarily elect true Condorcet winners when voters have polling information and then vote strategically. Black's, Copeland's, Dodgson's, and Kemeny's Condorcet-completion rules all fail this real-world test. a) Sincere Voting Interest groups' ballots Ballot 4 4 1 ranks voters voters voter 1st A B C 2nd B A A 3rd C C B Pairwise comparisons A gets 5 votes to 4 against B etc. A B C A wins - 5:4 8:1 B 4:5 - 8:1 C 1:8 1:8 - From this pre-election survey, the major voting rules give a unanimous result: Candidates Voting rule A B C Agenda v Plurality 4 4 tie Runoff v Approval 1 4 4 1 tie Approval 2 9 8 1 Black v Borda 13 12 2 Coombs v X Copeland 2 0 -2 C-IRV v Dodgson 0 -1 -4 M-IRV v X Kemeny 0 -1 -8 Minimax +11 -11 -78 Std-score[2] 4 3 -7 (Con) = a Condorcet-completion system. X = an eliminated candidate. v = victory. |
|
Now all voters know that A leads the race. Voters opposed to A can "punish" her with last-place votes to decrease her score relative to the other candidates. In Example 1 b, supporters of B decide to vote strategically. b) Strategic Voting by B's party Interest groups' ballots Ballot 4 4 1 ranks voters voters voter 1st A B C 2nd B C A 3rd C A B Pairwise comparisons. A gets 5 votes to 4 against B etc. A B C A - 5:4 4:5 B 4:5 - 8:1 C 5:4 1:8 - A bests B who bests C who bests A. This voting cycle makes the Condorcet-completion rules use a second rule to decide their winners. Whether or not they are based on Condorcet, most rules are easily defeated by punishing votes. Our rules produce these results for the final election: Candidates Voting rule A B C Approval 1 4 4 1 tie Approval 2 5 8 5 Black v Borda 9 12 6 Coombs X v X Copeland 0 0 0 tie C-IRV v X Dodgson -1 -1 -4 tie M-IRV v X Kemeny -2 -2 -5 tie Minimax % -11 -11 -77 tie Std-score 0 3 -3 By voting strategically, B's supporters would win or tie the election according to most voting rules. C's supporters also can vote insincerely against A. But they would only help B not C. A's supporters may try to counter B's strategy by punishing B. In that case all of these rules would choose C; the least-liked candidate would be the apparent Condorcet winner! The important point is that A would not need to counter B's strategy in this case under C-IRV, or M-IRV.[2] Frequency of manipulable electionsPunishing is one of the easiest ways for voters to manipulate an election.[4] How difficult is it to manipulate an election? How often can voters manipulate an election?[5] The first question requires a framework based in psychology and information science (degrees of insincerity, degrees of risk, amount or type of information needed, communication channels needed). The second question needs mathematical proofs or statistics from lifelike simulations. Chamberlin, Cohen, and Coombs assessed the minimum numbers of voters needed to change the winners of actual elections. They used the ballots from five elections for the presidency of the American Psychological Association.data from Chamberlin, Cohen, and Coombs (1984) The total number of voters increased over the years. Year 1976 1978 1979 1980 1981 # of voters 11560 15285 13535 15449 14223 |
Voting 1976 1978 1979 1980 1981 system U P U P U P U P U P Plurality 500 500 552 552 551 551 778 778 1 1 Borda 444 964 72 476 591 842 158 849 28 104 Hare (IRV) * * 35 * * * * * * * Coombs 834 1430 468 26 63 64 36 524 254 517 Approve 2 375 662 99 379 293 406 32 428 286 307 Approve 3 714 1199 454 740 373 705 868 1277 20 156 Kemeny 1312 1822 572 819 821 971 240 957 467 566 Minimax 1410 2110 575 801 783 1240 242 1006 467 566 Black 1200 1588 531 649 616 971 231 616 321 410 |
|
* = Manipulation not possible. (Con) = a Condorcet completion system. Approve 2 = Approval votes for the voter's top 2 choices. Approve 3 = votes for his top 3 choices. U = Uniform majority ordering P = Proportional majority ordering Uniform and proportional majority orderings were used to fill the empty ranks of ballots on which the voters marked only their first few choices. Uniform ordering filled-up ballots randomly so as to give no net advantage to any remaining candidate. "This corresponds to the assumption that voters are indifferent to candidates whom they do not rank." Proportional ordering made the artificially-completed ballots resemble voter-completed ballots with the same top preferences. This method corresponds to an assumption that voters omitted candidates because they lacked sufficient knowledge, and that if these voters had the knowledge necessary to complete their ballots they would have done so with the same preferences as those on the similar but complete ballots. More people must conspire to manipulate a proportionally-filled ballot set than are needed to manipulate the same ballots filled uniformly. with the Incentive and Ability to Aid in Manipulation |
Voting 1976 1978 1979 1980 1981 system U P U P U P U P U P Plurality 16.0 14.6 13.3 12.6 16.0 6.2 18.6 18.2 0.1 0.1 Borda 8.6 19.2 1.0 7.0 10.2 14.5 2.2 12.4 0.5 0.5 Hare(IRV) * * 0.7 * * * * * * * Coombs 16.2 28.2 6.8 0.4 1.1 1.1 0.5 7.9 4.0 8.2 Approve 2 12.6 21.3 2.7 9.5 14.2 21.2 0.8 10.5 11.0 13.1 Approve 3 21.2 33.4 11.6 23.1 14.1 26.5 27.3 39.4 0.6 4.5 Kemeny 27.7 46.4 8.1 12.1 14.1 16.7 3.5 15.0 18.9 22.4 Minimax 63.1 91.5 23.2 32.8 45.4 74.7 10.3 32.1 18.9 22.4 Black 25.4 35.2 7.6 9.6 10.6 16.7 3.3 9.6 13.0 16.2 |
|
The researchers reported: “The most striking result is the difference between the manipulability of the Hare system and the other systems. Because the Hare system considers only 'current' first preferences, it appears to be extremely difficult to manipulate. To be successful, a coalition must usually throw enough support to losing candidates to eliminate the sincere winner (the winner when no preferences are misrepresented) at an early stage, but still leave an agreed upon candidate with sufficient first-place strength to win. This turns out to be quite difficult to do. The authors contrast those strategies for 2 pages. As they and Merrill imply, the first preference is the rank most likely to be sincere on each ballot. The manipulability of the three Condorcet-completion rules (Kemeny, Minimax, and Black) proves that in each election a group of voters could create a voting cycle and also change a count such as the Borda used by Black's rule. Still, page 6 shows the need to create a cycle makes C-IRV even harder to manipulate than M-IRV because it increases the number of voters who must be organized into a conspiracy. Tideman's findings reportedly agree with these.(Merrill, page 70) He used data from "thermometer" surveys of voter opinions about the candidates for the 1972 and 1976 presidential nominations. It is worth noting that he found Dodgson's Condorcet-completion rule about as resistant to manipulation as Hare's (M-IRV) rule. But to manipulate Dodgson's rule needs less information than IRV requires about other voters' preference lists. So those who want to manipulate Dodgson can plan and coax voters into a simple strategy. Irrelevant alternativesThe winner under Condorcet's criterion cannot be changed by removing any other candidate(s), nor by introducing any less popular candidate(s). (Merrill, page 98) Political scientists would say no one can manipulate it by introducing irrelevant alternatives. Politicians rather easily can manipulate many elections under other voting systems by using this strategy. That means politicians can make the winner become a loser by introducing a candidate who is less popular than the former winner. Introducing irrelevant alternatives includes the strategy by which parties help start-up candidates on the opposite political wing to divide the opposition.This political trick is fairly simple and common. Table 4. Violations of Independence of Irrelevant Alternatives
Voting system Violation % Plurality 19 Runoff 10 Approval 9 Borda 7 Hare (M-IRV) 6 Coombs 1 Black (CW/Borda) 0.1 [ C-IRV 0.1 estimated III ] |
Case Study: Republicans Split Northern and Southern Democrats.In the late 1950's the U.S. House of Representatives considered a bill to increase federal funds for local schools. The Democratic Party favored the bill and had enough votes to pass it. Republicans, opposed to the bill, reasoned that if they proposed an amendment to block the funding of segregated schools, Northern Democrats would be compelled by constituents to support it. The Southern Democrats then would have no political choice but to join the Republicans in voting against the amended bill. The Northern and Southern Democrats behaved predictably and the Republicans succeeded in killing the school-funding bill.Let's see what would have happened under different voting procedures. Here are the approximate sizes and preferences of the three voting blocks. Example 2.
Ballot 161 Northern 80 Southern 160 ranks Democrats Democrats Republicans 1st Amended Bill No bill 2nd Bill No bill Amended 3rd No bill Amended Bill Pairwise comparisons Amended Bill Bill 80:321 - No bill 240:161 160:241 This is a voting cycle. The amended bill beats the plain bill by 321 votes to 80 votes. The plain bill beats no bill by 241 votes to 160. And no bill beats the amended bill 240 to 161. A > B > N > A. Should they pass a bill to increase funding and fight segregation? If the House votes first on funding then on desegregation both would pass; if the Republicans vote for the desegregation amendment they proposed. But most parliamentary procedures require voting on the amendment before the bill. So the House would pass the amendment and then defeat the bill - as actually happened. This case follows Duncan Black's rule of thumb as cited by Straffin, "...the later you bring up your favored alternative, the better chance it has of winning"(page 20) Here Bill which could beat No bill was itself beaten in the previous round by Amended bill. They get the same result, nothing, from the C-IRV and M-IRV voting systems as noted below. Keep in mind that without the amendment, the plain Bill would have passed by 241 Democratic votes to 160 Republican votes. Options Voting Amended Plain No Rule Bill Bill bill Agenda v Plurality 161 80 160 Runoff v Approve of 1 161 80 160 Approve of 2 321 241 240 Black v Borda 482 321 400 Chamberlin X v Coombs v X Copeland 0 0 0 tie C-IRV X v Dodgson -40 -121 -41 M-IRV X v Kemeny -40 -121 -41 Minimax % -19.7 -60 -20.2 |
|
If the Northern Democrats out-number the Republicans then Amended bill would win by most rules. Under Copeland all options would tie. Under agenda, M-IRV, and C-IRV No bill would win. We would say C-IRV was manipulated by an irrelevant alternative because the new alternative did not win, yet reversed the order of the original two options. The Republicans might argue that they exposed the fact that some of the school funds would have gone to support racist school districts which most voters did not approve of and did not want to pay taxes for. Because of this new issue dimension, previously unconsidered, C-IRV reverses its result. If the Republicans had added an amendment to set funding higher or lower than the Democrat's bill, then the C-IRV result would not be reversed. No bill would still be defeated - by either the original Bill or the Amended bill's funding amount. The amendment certainly was not an irrelevant issue; but strictly speaking it was an irrelevant alternative. 2 Issue Dimensions Funding $ Desegregation Yes 241 321 (maybe) No 160 80 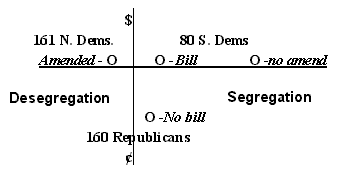 |
|
If the Republicans outnumber the Northern Democrats, that switch of one vote changes the result to No bill under most voting systems. If the Republicans rank the desegregation Amended bill last, and raise the plain Bill to second place, then the plain Bill would beat each of the other options in one-on-one contests and win under most voting systems. No bill could still win only under agenda and Hare. The Republicans in this case used several manipulation techniques. First they introduced an amendment that some theorists might consider an irrelevant alternative. It created a voting cycle. Then they probably voted insincerely to punish the leading option. No one can prove insincere votes but many of these same Republicans often voted against desegregation so I doubt they sincerely preferred the Amended bill over the plain Bill. I give this negative example of C-IRV last to impress upon readers that no decisive, non-dictatorial voting system can guarantee complete resistance to manipulation in all situations. C-IRV is most subject to manipulation in committee voting. Dennis Mueller writes in a section titled "Cycling", "Thus it would seem that when committees are free to amend the issues proposed, cycles must be an ever present danger." (page 64) If the amendments create a cycle, then C-IRV starts to eliminate proposals. It is hard to manipulate that process, but it is possible. Deleting less popular candidates can change the winner also. This test uses real-world ballots to measure a voting system's vulnerability to changes in the slate's minor candidates. Table 5. Violations of Subset Rationality
Voting 2 3 4 system Candidates Candidates Candidates Total Plurality 5 7 2 14 Borda 2 2 1 5 Hare (M-IRV) 2 2 0 4 Coombs 0 0 1 1 Approve 2 1 17 1 19 Approve 3 3 6 5 14 [ Condorcet 0 0 0 0 R.L.] |
|
The authors note that "Violations of this subset rationality condition when a single candidate is omitted seem most serious..." Hare was the only system with no violations when a single candidate was omitted. There were no cycles in the real-life elections and polls studied by these authors. Other research also finds cycles are not common. “It is notable that both data sets have voting cycles — the 913 ANES surveys have 4 cycles (0.44 percent), and the 20,087 ERS [Electoral Reform Society] elections have 476 cycles (2.37 percent). However, there are only 101 voting cycles (1.45 percent) among the 6,794 ERS elections with 21 or more voters, and only 6 voting cycles (0.68 percent) among the 883 ERS elections with 350 and more voters. Thus the frequency of voting cycles falls fairly quickly as the number of voters increases.” The Structure of the Election-Generating Universe by T. Nicolaus Tideman and Florenz Plassmann; 2010 Sensitivity to incomplete ballotsWe may need or want to use incomplete ballots - ones with some candidates not ranked. Unfortunately all vote-counting rules will miss the most central candidate more often when voters cast incomplete ballots. Some systems will stumble due to a small percentage of bad ballots while other systems probably tolerate this problem better.To better understand the effects of incomplete ballots, we need a study similar Chamberlin and Cohen's on the deletion of candidates, shown in Table 5. For now I shall re-use some of their published results to estimate the sensitivity to incomplete ballots for five voting systems. Tables 2a and 2b in their article showed how five election rules ranked all candidates, from winner to last-place loser. Table 2a showed their results when they filled the incomplete ballots uniformly - giving no favor to any candidate. The researchers state: "This corresponds to the assumption that voters are indifferent to candidates whom they do not rank." Table 2b gave their results when they filled the ballots proportionally - making the artificially-completed ballots resemble voter-completed ballots with the same initial preferences. This method corresponds to an assumption that voters omitted candidates because they lacked sufficient knowledge, and that if these voters had the knowledge necessary to complete their ballots they would have done so with the same preferences as those on the similar but completed ballots. If a voting system showed many differences between those two tables, then it is very sensitive to how the incomplete ballots are filled - and probably sensitive to the use or deletion of incomplete ballots. Table 6 shows the number of differences, in winners and complete social rankings, between Chamberlin, and Cohen's Tables 2a and 2b.
Table 6. Sensitivity to Methods of Filling Incomplete Ballots
|
Ordering Generated by . Plural. Borda M-IRV Coombs App 2 App 3 Winners changed 0 1 1 1 0 0 Other positions " 0 1 1 6 1 4 |

|
|



 Accurate Democracy
Accurate Democracy